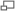I have forgotten
my Password

Or login with:

  • Facebookhttp://facebook.com/
  • Googlehttps://www.google.com/accounts/o8/id
  • Yahoohttps://me.yahoo.com
advanced search

Valid RSS

Engineering › Fluid Mechanics › Machines › Turbines ›

Axial Flow Turbines

An analysis of Axial Flow Turbines using both the Euler and Aerofoil Theories
+ View other versions (4)
Contents
  1. Introduction
  2. The Euler Theory
  3. The Aerofoil Theory Used For Turbines
  4. Page Comments

Introduction

Almost all electrical power on Earth is produced with a turbine of some type. Very high efficiency steam turbines harness about 40% of the thermal energy, with the rest exhausted as waste heat.

Most jet engines rely on turbines to supply mechanical work from their working fluid and fuel as do all nuclear ships and power plants.

Axial Turbine

An axial turbine operates in the reverse of an axial compressor. A set of static guide vanes or nozzle vanes accelerates and adds swirl to the fluid and directs it to the next row of turbine blades mounted on a turbine rotor.

A turbine is a rotary engine that extracts energy from a fluid flow and converts it into useful work.

MISSING IMAGE!

23287/Axial-Flow-Turbines-001.png cannot be found in /users/23287/Axial-Flow-Turbines-001.png. Please contact the submission author.

As with a Pump consider an element at radius \inline r.

MISSING IMAGE!

23287/Axial-Flow-Turbines-002.png cannot be found in /users/23287/Axial-Flow-Turbines-002.png. Please contact the submission author.

v = v_1 = \frac{2\pi RN}{60}

Q = \pi \left(R_0^2 - R_{Boss}^2 \right)V_f

V_1 = V_{f1} = V_f

The Euler Theory

Leonhard Euler (15 April 1707 - 18 September 1783) was a pioneering Swiss mathematician and physicist. He made important discoveries in fields as diverse as infinitesimal calculus and graph theory.

Work done by the water on the Vanes per lb. \inline = \displaystyle\frac{V_w\,v}{g} ( It is assumed that there is no whirl at exit).

Applying Bernoulli's equation across the Vanes and assuming no losses:

\frac{p}{w} + \frac{V^2}{2g} = \frac{p_1}{w} + \frac{V_1^2}{2g} + \frac{V_w\,v}{g}

\frac{p - p_1}{w} = \frac{V_w\,v}{g} + \frac{V_1^2 - V^2}{2g}

But since:
V^2 = V_w^2 + V_f^2 = V_w^2 + V_1^2

\frac{p - p_1}{w} = \frac{V_w\,v}{g} - \frac{V_w^2}{2g}

Hydraulic \inline \eta = \displaystyle\frac{V_w,v}{gH}

The Aerofoil Theory Used For Turbines

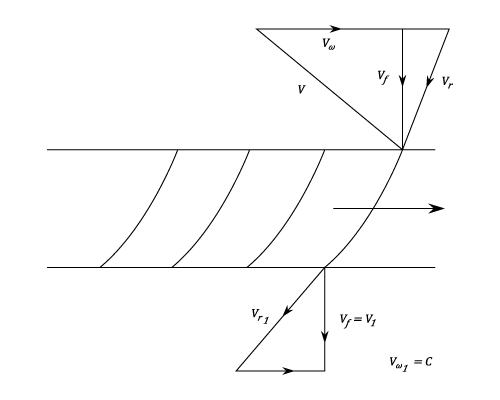
Combining the Velocity Triangles: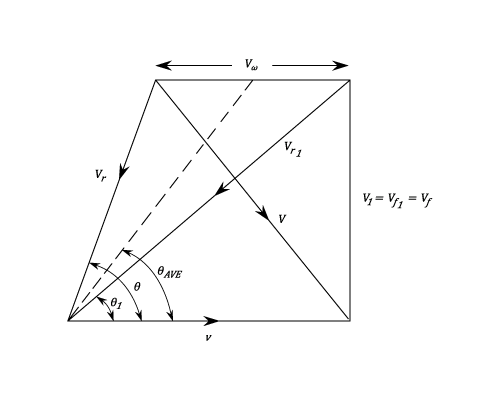

MISSING IMAGE!

23287/Axial-Flow-Turbines-003.png cannot be found in /users/23287/Axial-Flow-Turbines-003.png. Please contact the submission author.

The following diagram is a sketch through one of the Vanes:
MISSING IMAGE!

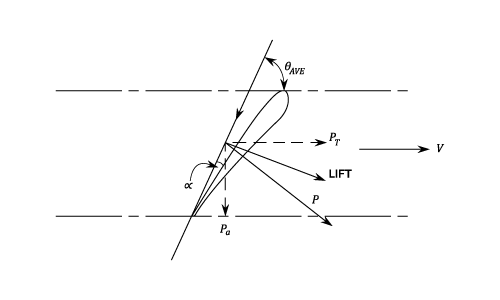
23287/Axial-Flow-Turbines-004.png cannot be found in /users/23287/Axial-Flow-Turbines-004.png. Please contact the submission author.

Consider the Vanes as Aerofoils in a fluid stream of Velocity \inline V_{r\,ave.} in a direction \inline \theta_{ave}.

For an elemental thickness \inline dR at a radius \inline R.


Lift \inline L
L = C_L\times \frac{1}{2}\;\rho\; V_{r\,ave.}^2\times C\times dR\;\;\;lbs.

Drag \inline D
D = C_D\times \frac{1}{2}\;\rho\; V_{r\,ave.}^2\times C\times dR\;\;\;lbs.

(where \inline C= Chord)
The vector sum of \inline L and \inline D is the resultant force \inline P on the Vane element.


The components of \inline P in the tangential Direction is given by:

P_t = L\;Sin\,\theta _{ave} - D\;Cos\,\theta _{ave.}\;\;\;lbs.

And the Axial component is :

P_a = L\;Cos\,\theta _{ave} + D\;Sin\,\theta _{ave.}\;\;\;lbs.

For \inline n Vanes:



The Total Tangential Force = \inline n Pt



The Torque \inline T = n Pt X R



The Horse Power output
= \frac{2\pi NT}{550\times 60}

Total Axial Force \inline = n Pa = The Thrust on the Bearings.
Last Modified: 29 Sep 11 @ 08:48     Page Rendered: 2023-01-22 22:34:07