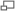Transmission of Power
The transmission of Hydraulic Power through pipelines. Includes Nozzles and Jets, the conditions for maximum Power Transmission , Jet reaction and the efficiency of transmission.
Contents
Introduction
The transmission of power through pipes is both common and widespread in its application. Two examples are the large diameter pipes are used in many hydroelectric schemes and the comparatively small bore pipes used to connect the various pumps; motors and rams used on earth moving plant and machine tools.
A diameter of a circle is any straight line segment that passes through the center of the circle and whose endpoints are on the circle.
Volume is the quantity of three-dimensional space enclosed by some closed boundary.
Power is the rate at which work is done, expressed as the amount of work per unit time and commonly measured in units such as the watt and horsepower.
Volume is the quantity of three-dimensional space enclosed by some closed boundary.
Power is the rate at which work is done, expressed as the amount of work per unit time and commonly measured in units such as the watt and horsepower.
Water Horse Power is given by:
 Weight of water / second =
Weight of water / second =  Horse Power of a jet is:
The efficiency of power transmission for the pipe is given by Horse Power at outlet/Horse Power at inlet which is:
Horse Power of a jet is:
The efficiency of power transmission for the pipe is given by Horse Power at outlet/Horse Power at inlet which is:
 Applying Bernoulli's equation to the pipe and ignoring pipe entry losses:
Thus the efficiency of Power Transmission can be written as:
Applying Bernoulli's equation to the pipe and ignoring pipe entry losses:
Thus the efficiency of Power Transmission can be written as:

The Conditions For Maximum Power Transmission
i.e. The head lost due to friction is one third of the Total Supply Head.From equations (2) and (3) the Horse Power of the Jet will be:
The weight of water per second,  , passing down the pipe increases linearly with
, passing down the pipe increases linearly with  , the velocity of the water along the pipe. However
, the velocity of the water along the pipe. However ) decreases with velocity and there is therefore an optimum velocity which will give the maximum jet Horse Power for a given pipe and Head.
Equation (4) can be rewritten using equation (1).
decreases with velocity and there is therefore an optimum velocity which will give the maximum jet Horse Power for a given pipe and Head.
Equation (4) can be rewritten using equation (1).  is replaced using the Darcy equation:
is replaced using the Darcy equation:
)
) For Maximum Power
For Maximum Power  i.e.
i.e.
=0) From which it can be seen that
From which it can be seen that  i.e. The head lost due to friction is one third of the Total Supply Head.
i.e. The head lost due to friction is one third of the Total Supply Head.
The Efficiency Of Power Transmission For Maximum Power
- This is given by:
The condition for Maximum Power transmitted is rarely used in practice since:
- The efficiency of transmission is low and that means that water is wasted;
- The velocity in the pipeline is high and this can give rise to dangerous Water Hammer effects when valves are closed.
Nozzles
A nozzle is a device designed to control the direction or characteristics of a fluid flow as it exits an enclosed chamber or pipe via an orifice.
The Nozzle Diameter For Maximum Power Transmission
In the following calculations the losses in the nozzle are ignored. Two proofs are given. The first uses the previous result for the power transmission through a Pipe Line and the second is from first principles.
Diagram from notes.
 Or:
Or:


^2=\frac{8fl}{d}) But:
But:
 Or:
Or:
^2)
^4=\frac{8fl}{D}) Thus:
Thus:

 And using the continuity equation:
And using the continuity equation:
^4\times&space;\frac{v^2}{2g}+\frac{v^2}{2g}=&space;\frac{v^2}{2g}\left&space;[&space;\frac{4fl}{D}\left&space;(&space;\frac{d}{D}&space;\right&space;)^4+1&space;\right&space;])
^4}+1}) The power output of the nozzle is given by:
The power output of the nozzle is given by:
^4}+1&space;\right&space;)^{\frac{3}{2}})
^4+1&space;\right&space;)^{-\frac{3}{2}}) The nozzle diameter for the maximum power transmission will occur when
The nozzle diameter for the maximum power transmission will occur when }=0) i.e.
i.e.
^4+1&space;\right&space;]^{-\frac{3}{2}}=\frac{3}{2}d^2\left&space;[&space;4fl\left&space;(&space;\frac{d}{D}&space;\right&space;)^4+1&space;\right&space;]^{-\frac{5}{2}}\times&space;4\times&space;\frac{4fl}{D}\times&space;\frac{d^3}{D^4}) Multiply both sides by
Multiply both sides by
^4+1&space;\right&space;]^{\frac{5}{2}})
^4+1=\frac{3}{2}\times&space;\frac{d\times&space;2\times&space;4fld^3}{D^5}=\frac{12\times&space;fl}{D}\left&space;(&space;\frac{d}{D}&space;\right&space;)^4) Or:
Or:
^4=1)

- a) It has been shown that the maximum Power transmission through a pipe lie occurs when the head lost due to friction is a third of the total head.
Hence:
- b) from first principles
Jet Reaction
The jet reaction is the backward force exerted on the nozzle and the pipe and is equal and opposite to the force exerted by the jet on a fixed plate. i.e. The jet reaction = the mass of fluid passing through the jet per secondTherefore the Jet Reaction will be
The Efficiency Of Power Transmission For Maximum Jet Reaction
- The efficiency,
Example:
[imperial]
Example - Example 1
Problem
A Nozzle discharges 175 galls per min. under a head of 200 ft. The diameter of the nozzle is 1 in.
and the diameter of the jet is 0.9 in.
Find:
- The coefficient of velocity for the jet.
- The head lost in the nozzle.
- The horse power available in the jet.
Workings
- a) The Coefficient of Contraction,
is given by:
- b) Using Equation (5)
- c) The horse power of the jet is dependent upon the weight of water per second and the head of water in the jet
Thus:
Horse power will be:
Solution
- a) The coefficient of velocity is
- b) The head lost in nozzle is
- c) The horse power available in the jet is
 Login
Login