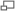Engineering › Thermodynamics ›

The Thermodynqmics of a Perfect Gas

Examines Boyle's; Charles's; Joule's; Dalton's Laws and details Specific heat of Gasses

View other versions (3)

Contents

The Thermodynamics Of A Perfect Gas.
Boyle's Law.
Charles's Law.
The General Gas Equation.
The Characteristic Gas Equation.
Pound-mols
Avrogadro's Hypothesis
The Universal Gas Equation.
Joule's Law Of Internal Energy
The Specific Heats Of A Perfect Gas.
The Molecular Specific Heat:-
The Enthalpy Of A Perfect Gas.
The Ratio Of Specific Heats:-
Summary
Page Comments

The Thermodynamics Of A Perfect Gas.

A Perfect Gas is a Working Substance which obeys the Following:

Boyle's Law
Charles's Law
Joule's Law of internal Energy
Dalton's Law of Partial Pressures
Has a Specific heat which is Constant

To obey all these laws the substance would:

Not be able to change it's state even at Absolute Zero
The molecules would need to be so far apart that there are no inter molecular forces and no collisions

At normal Temperature and Pressures the Permanent gases closely obey these Laws. E.g. Oxygen; Nitrogen; Helium etc. These gases are called "Semi-Perfect"

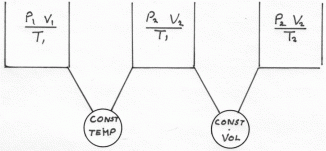
Boyle's Law.

If the Temperature remains constant,. The Pressure of a fixed mass of gas is inversely proportional to it'sVolume.

$With\;T\;constant\;\;\;\;\;PV = Constant$

(2)

Charles's Law.

If the Volume of a fixed mass of gas is kept Constant, then it's pressure is directly proportional to it's Absolute temperature

$With\;V\;Constant\;\;\;\;\;\frac{P}{T} = Constant$

(3)

The General Gas Equation.

MISSING IMAGE!

13108/img_th10.jpg cannot be found in /users/13108/img_th10.jpg. Please contact the submission author.

$For\;Isothermal\;Conditions\;\;\;\;\;P_1V_1 = P_2V_2$

(4)

$For\;Isovolumic\;conditions\;\;\;\;\;\frac{P_1}{T_1} = \frac{P_2}{T_2}$

(5)

$Dividing\;\;\;\;\;\;\;\;\frac{P_1V_1}{T_1} = \frac{P_2V_2}{T_2}$

(6)

The Characteristic Gas Equation.

$\frac{PV}{T} = Constant$

(7)

Consider w lbs of Gas:-

$\frac{PV}{wT} = Constant = R\;\;\;(The \;characteristic\; Gas\; Constant)$

(8)

$\therefore\;\;\;\;\;PV = wRT$

(9)

The Units of R (Imperial)

$= \frac{lb.ft.^3}{ft^2}\times \frac{1}{lb}\times \frac{1}{^0F} = ft.lb/lb^0F$

(10)

The Characteristic Constant for Air ( Imperial):-

1lb.of air at 32^0F and 14.7 psi occupies 12.39 cu.ft.

$R_{air} = \frac{PV}{wT} = \frac{14.7\times 144\times 12.39}{1\times 492}\;\;\;\;\;(32^0F = 492^0R)$

(11)

$= 53.3\;ft.lb./lb.^0F = 96ft.lb./lb.^0C = \frac{53.3}{778}\;BTU/lb.^0F$

(12)

Pound-mols

The Lb-Mol is a measure of weight and equals the molecular weight of a gas expressed in pounds.

One Lb-Mol of Oxygen weighs 32 Lbs.

One Lb-Mol of Nitrogen weighs 28 Lbs.

One Lb-Mol of $\inline CO_2$ wieghs 44 lbs.

Avrogadro's Hypothesis

This states that equal volumes of gas at the same temperature and Pressure contain the same number of Molecules. This means that one Lb-Mol of ANY gas will occupy the same Volume at the same temperature and Pressure.

One lb-Mol at 14.7 psi and at $\inline 32^0F$ occupies 358 cu.ft.

This also means that Proportions by Volume are the same as Proportions by Mols.

The Universal Gas Equation.

The universal gas equation ties together pressure, volume and temperature:

$\frac{PV}{T}= wR$

(13)

where R is the universal gas constant.

$\frac{P}{T}\times \frac{V}{w} = R$

(14)

$\therefore\;\;\;\;\;\frac{Pv}{T} = R\;\;\;\;\;\;where\;v\; is\;the\;volume\;of\;one\;lb.$

(15)

Multiply both sides by the Molecular weight M

$\therefore\;\;\;\;\;\frac{PMv}{T} = MR\;\;\;\;\;\;Mv\;is\;the\;Volume/lb.mol.$

(16)

Note. Mv is a constant for ANY gas at a given temperature and Pressure.

Therefore MR is also a Constant for any gas at a given temperature and Pressure.

MR is called the universal gas constant and is given the symbol $\inline \tilde{R}$

$\therefore\;\;\;\;\;P(mv) = \tilde{RT}$

(17)

$\therefore\;\;\;\;\;P(\frac{V}{N}) = \tilde{RT}\;\;\;\;\;Where\;N = the\;number\;of\;lb.mols$

(18)

$\therefore\;\;\;\;\;PV = N\;\tilde{R}T$

(19)

This Equation is known as theUniversal Gas Equation

The Units of the Universal Gas Constant are ft.lbs/Mol Degree Rankin.

$One\;Mol.\;of\;any\;gas\;at\;32^0F\;and\;14.7\;psi\;occupies\;358\;ft^3$

(20)

Joule's Law Of Internal Energy

Joule's Law states that for a PERFECT gas the Internal Energy is independent of the Pressure and Volume and depends only on the Temperature of the Gas.

$i.e.\;\;\;\;\;\;E\;is\;a\;function\;of\;the\;Absolute\;Temperature$

(21)

The Specific Heats Of A Perfect Gas.

The Specific heat is the quantity of heat required to raise one pound of a substance through a temperature rise of one degree.

$q = \delta\,E + w$

(22)

In the case of gases the Specific Heat depends upon the way in which the gas is heated. i.e. If it is allowed to do work the Specific Heat must be greater. There are therefore an infinite number of Specific Heats but only two will be considered here.

Specific heat at Constant Volume. (C_V).
Specific heat at Constant Pressure. (C_p)

Consider a heating process at Constant Volume.

$q = \delta\,E + pdV$

(23)

$q = \delta\,E = w\,C_V(T_2 - T_1)$

(24)

since

$E = fT$

(25)

Then $\inline \delta\,E$ is the same for any process and equals:-

$\delta\,E = w\,C_V(T_2 - T_1)$

(26)

If the Absolute Zero is taken as the Datum. $\inline E = w\,C_V\,T$

Consider a heating process at Constant Pressure.

$q = \delta\,E + p\,dV$

(27)

$\therefore\;\;\;\;\;q = w\,C_p(T_2 - T_1)$

(28)

$p\,dV = p(V_2 - V_1) = p_2(V_2 - V_1)\;\;\;\;\;since \;p_1 = p_2 = p_0$

(29)

$= wR(T_2 - T_1)$

(30)

Substituting in the First Law:

$wC_P(T_2 - T_1) = wC_V(T_2 - T_1) + wR(T_2 - T_1)$

(31)

$C_P = C_V + R\;\;\;\;\;\;or\;\;\;\;\;R = C_P - C_V$

(32)

The Molecular Specific Heat:-

This is the heat required to raise one pond mol. of a substance through one degree.

$\inline _nC_P$ is the specific heat at constant Pressure ( $\inline C_p$ ) times Molecular weight

$\inline _nC_V$ is the specific heat at constant Volumen ( $\inline C_V$ ) time Molecular weight

$\therefore\;\;\;\;\;\tilde{R} = _nC_P - _nC_V\;\;\;\;\;(BTU/lb.mol.\,^0F)$

(33)

The Enthalpy Of A Perfect Gas.

$H_2 - H_1 = E_2 - E_1 + P_2V_2 - P_1V_1$

(34)

$= wC_V(T_2 - T_1) + wR(T_2 - T_1)$

(35)

$= w(T_2 - T_1)(C_V + R) = wC_P(T_2 - T_1)$

(36)

Using the Absolute Datum as the Datum, then H = 0 when T = 0

$H = w\,C_P\,T$

(37)

The Ratio Of Specific Heats:-

$let\;\;\;\;\;\gamma = \frac{C_P}{C_V}$

(38)

The value of $\inline \gamma$ varies depending upon Atomic Conditions. One Atom can only rotate; Two can each rotate about their own axis and they can rotate about each other.