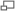Ellipse

Analysis of the Ellipse; Its Tangents and the Auxiliary Circle Chords

View other versions (2)

Contents

Definition
Ellipse General Equation
The Tangent To An Ellipse At A Given Point
The Normal To The Tangent At (x',y')
The Points Of Intersection Of A Straight Line And An Ellipse
The Parametric Equation Of An Ellipse
The Parametric Equation Of A Chord
The Equation Of A Tangent
The Meet Of Two Tangents At Points With Differing Eccentric Angles
Page Comments

Definition

An ellipse is the locus of all points of the plane whose distances to two fixed points add to the same constant.

An ellipse can be obtained as a result from the intersection of a cone by a plane in a way that produces a closed curve. Circles are special cases of ellipses, obtained when the cutting plane is orthogonal to the cone's axis.

Ellipse General Equation

If X is the foot of the perpendicular from S to the Directrix, the curve is symmetrical about the line XS. This line is taken to be the x axis.

The ratio $\inline e=\frac{SP}{PK}$ ,is called eccentricity and is less than 1 and so there are two points on the line SX which also lie on the curve.
One A' will lie between between S and X and nearer S and the other X will lie on XS produced.
Let the distance AA' be 2a and let the mid point of AA' be the point C.
C is called the Centre of the Ellipse and is taken to be the origin.

The eccentricity of an ellipse is :

$e=\varepsilon=\sqrt{\frac{a^2-b^2}{a^2}}$

MISSING IMAGE!

22109/ellipse_1.gif cannot be found in /users/22109/ellipse_1.gif. Please contact the submission author.

Since A lies on the curve:

$SA = e*AX$

Since A' lies on the curve:

$SA' = e*A'X$

Adding

$SA + SA' = e(AX + A'X)$

But

$AX + A'X = a + CX + CX\;-a = 2a$

(2)

Therefore $\inline 2a = e(2\,CX)$ or $\inline CX = \frac{a}{e}$

Also

$CS = a - A'S = a - eA'X = a - e\left(\frac{a}{e} - a \right) = ae$

(3)

We now the lengths of both CX and CS and can proceed to find the equation of the curve.

from equation (3) we know that $\inline CS = ae$

The area of an ellipse is :

$A=\pi a b$

This result can be proven by direct integration.

Therefore

$PS^2 = (x + ae)^2 + y^2$

(4)

and

$PK = x + CX\;=x + \frac{a}{e}$

(5)

also $\inline SP = e\;PK$

Therefore

$(x + ae)^2 + y^2 = \left[e\left(x + \frac{a}{e} \right) \right]^2$

(6)

$= (ex + a)^2$

(7)

Therefore

$x^2(1 - e^2) + y^2 = a^2(1 - e^2)$

(8)

$\frac{x^2}{a^2} + \frac{y^2}{a^2(1 - e^2)} = 1$

(9)

Let $\inline b^2 = a^2(1\,-\,e^2)$ and the equation of the Ellipse becomes:

$\mathbf{\frac{x^2}{a^2} + \frac{y^2}{b^2} = 1}$

(10)

The perimeter of an ellipse can't be expressed in a simple form. One approximation for the perimeter is $\inline p=\sqrt{2(a^2+b^2)}$ .

The length a is called the semi-major axis. When $\inline x = 0$ $\inline y$ is plus or minus b which is called the semi-minor axis.

Notes

The equation of an ellipse if the axis of length 2a is taken up the y axis is given by:

$\mathbf{\frac{x^2}{b^2} + \frac{y^2}{a^2} = 1}$

The major axis is still 2a. i.e. The major axis is always the larger of the two.

The equation of an Ellipse referred to a parallel axes through the point $\inline (h,k)$ is given by the equation:

$\mathbf{\frac{(x - h)^2}{a^2} + \frac{(y - k)^2}{b^2} = 1}$

Example:

Example - Focal distances

Problem

Show that the sum of the focal distances of any point on an is Ellipse is equal to the length of the major axis. Deduce a simple mechanical method for constructing the curve

Workings

From the diagram and from the definition of an ellipse, if $x$ is the abscissa of $P$

$PS = e\,PM = e\left(\frac{a}{e} + x \right) = a + ex$

Similarly if $PM'$ is drawn perpendicularly to the second Directerix $A'B'$

$PS' = e\,PM' = e\left(\frac{a}{e} - x \right) = a - ex$

The sum of the focal distances $PS$ and $PS'$ is therefore equal to $2a$ , the length of the major axis.

Solution

By fixing two pins at $S$ ans $S'$ and keeping stretched, by a pencil point, an endless piece of string passing round the pins, the pencil will describe an ellipse with $S$ , $S'$ as foci.

The Tangent To An Ellipse At A Given Point

Differentiating the equation for an ellipse with respect to $\inline x$

$\frac{2x}{a^2} + \frac{2y}{b^2}\frac{dy}{dx} = 0$

Hence the gradient at $\inline (x_1,y_1)$ is $\inline \displaystyle\left(\frac{dy}{dx} \right)_{x+x_1}\;= - \frac{b^2x_1}{a^2y_1}$

The equation of the tangent at $\inline (x_1,y_1)$ is therefore given by:

$y - y_1\;= - \left(\frac{b^2x_1}{a^2y_1} \right)(x - x_1)$

This can be written as:

$b^2xx_1 + a^2yy_1 = b^2x_1^2 + a^2y_1^2$

Dividing through by $\inline a^2b^2$ and using the equation for an ellipse,which is the condition that the point $\inline (x_1,y_1)$ shall lie on the ellipse, the equation of the tangent at the point $\inline (x_1,y_1)$ can be written as:

$\mathbf{\frac{xx_1}{a^2} + \frac{yy_1}{b^2} = 1}$

(11)

The Normal To The Tangent At (x',y')

The Normal at the point $\inline (x_1,y_1)$ is the line perpendicular to the tangent and therefore its slope is $\inline \displaystyle\frac{a^2y_1}{b^2x_1}$ and its equation is:

$y - y_1 = \left(\frac{a^2y_1}{b^2x_1} \right)(x - x_1)$

Example:

Example - Normal and Tangent

Problem

Find the equation of the Tangent and Normal to the Ellipse $5x^2 + 3y^2 = 137$ at the point in the first quadrant whose ordinate is $2$ .

Workings

The abscissae of points on the ellipse at which the ordinate is $2$ are given by putting $y = 2$ in the equation of the ellipse. This gives $5x^2 = 137 - 3(2)^2$ i.e. $x = \pm 5.$ . The point in the first quadrant with these coordinates is $(5,2)$ The equation of the ellipse can now be written in the form:

$\frac{x^2}{137/5} + \frac{y^2}{137/3} = 1$

Solution

Thus $a^2 = 137/5$ and $b^2 = 137/3$
The equation of the tangent is thus given by:

$\frac{5x}{137/5} + \frac{2y}{137/3} = 1$

The equation of the Normal is given by the equation

$\frac{x - 5}{5/(137/5)} = \frac{y - 2}{2/(137/3)}$

The Points Of Intersection Of A Straight Line And An Ellipse

The coordinates of the points of intersection of the straight line $\inline y = mx + c$ and the ellipse $\inline (x^2/a^2) + (y^2/b^2) = 1$ are the values of x and y which simultaneously satisfy both equations. Writing $\inline y = mx + c$ in the equating of the ellipse:

$\frac{x^2}{a^2} + \frac{(mx + c)^2}{b^2} = 1$

$(a^2m^2 + b^2)x^2 + 2a^2mcx + a^2(c^2 - b^2) = 0$

This quadratic has real, equal or imaginary roots depending upon whether $\inline c^2$ is positive,zero or negative. When $\inline c^2 < a^2m^2 + b^2$ , the line intersects the ellipse in two real roots. When $\inline c^2\;>\;a^2m^2 + b^2$ , the line intersects the ellipse only in imaginary points and when $\inline c^2 = a^2m^2 + b^2$ the line is a tangent to the ellipse.

Writing $\inline c = \sqrt{a^2m^2 + b^2}$ in the equation of the line:

$y = mx + \sqrt{a^2m^2 + b^2}$

we get a line which always touches the ellipse. Also since the radical sign on the right hand side of the equation may be either positive or negative, there are two tangents parallel to each other.

Example:

Example - Locus of points

Problem

Find the locus of the points of intersection of tangents to an ellipse which are at right angles to each other.

Workings

Using the standard equation for a Ellipse:

$\frac{x^2}{a^2} + \frac{y^2}{b^2} = 1$

And the equation for a tangent from above:

$y = mx + \sqrt{a^2m^2 + b^2}$

The equation of a perpendicular tangent at a gradient of $-1/m$ is :

$y\;= - \frac{1}{m}x + \sqrt{\left(\frac{a^2}{m^2} + b^2 \right)}$

These two equations for perpendicular tangents to the ellipise can be written as:

$y - mx = \sqrt{(a^2m^2 + b^2)}$

and $my + x = \sqrt{(a^2 + b^2m^2)}$

The coordinates of the point of intersection of the tangents simultaneously satisfy these two equations. If m is eliminated from the two equations the locus of the pint of intersection will be found.

Squaring and adding:

$(1 + m^2)(x^2 + y^2) = a^2m^2 + b^2 + a^2 + b^2m^2$

$x^2 + y^2 = a^2 + b^2$

Solution

The locus is therefore a circle with centre coincident with the centre of the ellipse and with a radius of $\sqrt{a^2 + b^2}$ . This circle is called the director circle.

The Parametric Equation Of An Ellipse

It is often convenient to express the coordinates of any point on the ellipse in terms of one variable.

Draw a circle on the major angle of an ellipse with a centre coinciding with the centre of the ellipse. This circle is called The Auxiliary Circle

MISSING IMAGE!

22109/ellipse_2.gif cannot be found in /users/22109/ellipse_2.gif. Please contact the submission author.

Draw a radius $\inline CQ$ of this circle making an angle $\inline \theta$ with the major axis. Then $\inline CQ = a$ and the coordinates of $\inline Q$ are $\inline (a\;cos\,\theta \;and\lb\,\;sin\,\theta$ .Draw the perpendicular from $\inline Q$ to the major axis to meet the ellipse at $\inline P$ . The $\inline x$ -coordinate of $\inline P$ is also $\inline a\;cos\,\theta$ and since the point $\inline P$ lies on the ellipse:

$\frac{x^2}{a^2} + \frac{y^2}{b^2} = 1$

$cos^2\;\theta + \frac{y^2}{b^2} = 1$

Therefore $\inline y^2 = b^2(1 - cos^2\;\theta )\:=\:b^2sin^2\;\theta$ Thus $\inline y = \pm\; b\;sin\;\theta$

Therefore the Parametric equation of an ellipse is :

$x = a\;cos\;\theta$

$y= b\;sin\;\theta$

The Parametric Equation Of A Chord

The gradient of the chord joining the points $\inline (a\;cos\,\alpha , b\;sin\,\alpha)$ and $\inline (a\;cos\,\beta , b\;sin\,\beta )$ is given by:

$\frac{b(sin\,\beta - sin\,\alpha ) } {a(cos\,\beta - cos\,\alpha )} = \frac{2b\;cos\,\frac{1}{2}(\alpha + \beta )\;sin\;\frac{1}{2}(\beta - \alpha )}{2a\;sin\frac{1}{2}(\alpha + \beta )\;sin\frac{1}{2}(\alpha - \beta )}$

$= - \frac{b\;cos\;\frac{1}{2}(\alpha + \beta )}{a\;sin\;\frac{1}{2}(\alpha + \beta )}$

$\frac{y - b\;sin\;\alpha }{x - a\;cos\;\alpha }= - \frac{b\;cos\;\frac{1}{2}(\alpha + \beta )}{a\;sin\;\frac{1}{2}(\alpha + \beta )}$

$\therefore\;\;\;\;\;ay\;sin\frac{\alpha + \beta }{2} + bx\;cos\;\frac{\alpha + \beta }{2} = ab\left(sin\;\alpha \;sin\;\frac{\alpha + \beta }{2} + cos\;\alpha \;cos\;\frac{\alpha + \beta }{2} \right)$

$= ab\;cos\;\frac{\alpha - \beta }{2}$

The Equation of the Chord is therefore

$\mathbf{\frac{x}{a}\;cos\;\frac{\alpha + \beta }{2} + \frac{y}{b}\;sin\;\frac{\alpha + \beta }{2} = cos\;\frac{\alpha - \beta }{2}}$

The Equation Of A Tangent

If $\inline \beta = \alpha$ the above equation for a Chord becomes the equation of a Tangent at the point $\inline (a\;cos\;\alpha,\;b\;sin\alpha)$ .

The equation is:

$\mathbf{\frac{x\;cos\;\alpha }{a} + \frac{y\;sin\;\alpha }{b} = 1}$

The Meet Of Two Tangents At Points With Differing Eccentric Angles

Let the two points have eccentric angles of $\inline \alpha$ and $\inline \beta$ . Therefore the equations of the tangents are:

${\frac{x\;cos\;\alpha }{a} + \frac{y\;sin\;\alpha }{b} = 1}$

and

$\frac{x\;cos\;\beta }{a} + \frac{y\;sin\;\beta }{b} = 1$

Eliminating $\inline y$ from these equations:

$\frac{x}{a}(cos\,\alpha \;sin\,\beta - cos\,\beta \;sin\,\alpha ) = sin\,\beta - sin\,\alpha$

Therefore

$\frac{x}{a}\;sin\;(\beta - \alpha ) = 2\;sin\frac{1}{2}(\beta - \alpha )\;cos\;\frac{1}{2}(\beta + \alpha )$

$\frac{x}{a}\times2\;sin\frac{1}{2}(\beta - \alpha )\;cos\;\frac{1}{2}\;(\beta - \alpha ) = 2\;sin\frac{1}{2}(\beta - \alpha )\;cos\;\frac{1}{2}(\beta + \alpha )$

Therefore

$x = a\;\frac{sin\;\frac{1}{2}(\alpha + \beta )}{cos\;\frac{1}{2}\;(\beta - \alpha )}$

Eliminating $\inline x$ from the equations:

$\frac{y}{b}\;(sin\,\alpha \;cos\,\beta - sin\,\beta \;cos\,\alpha ) = cos\,\beta - cos\,\alpha$

Therefore

$\frac{y}{b}\;sin\,(\alpha - \beta ) = 2\;sin\,\frac{1}{2}(\alpha - \beta )\;sin\,\frac{1}{2}\,(\alpha + b)$

Thus

$y = b\;\frac{sin\,\frac{1}{2}(\alpha + \beta )}{cos\,\frac{1}{2}(\alpha - \beta )}$

But since $\inline cos\,\frac{1}{2}(\alpha - \beta ) = cos\,\frac{1}{2}(\beta - \alpha )$ the point of intersection of the Tangents is:

$\left(a\;\frac{cos\,\frac{1}{2}(\alpha + \beta }{cos\,\frac{1}{2}(\alpha - \beta )}\;,\;b\;\frac{sin\,\frac{1}{2}(\alpha + \beta )}{cos\,\frac{1}{2}(\alpha - \beta )} \right)$

Example:

Example - Chord

Problem

The Chord $PQ$ of an Ellipse passes through the focus $(ae,0)$ . Show that the meet of the tangents at $P$ and $Q$ lies on the Directrix $\displaystyle x = \frac{a}{e}$ .

Workings

Suppose $P = (a\,cos\,\alpha \;,\;b\,sin\,\alpha)$ and $Q = (a\;cos\,\beta \;,\;b\,sin\,\beta )$
The equation of $PQ$ is :

$\frac{x}{a}\;cos\,\frac{1}{2}(\alpha + \beta ) + \frac{y}{b}\,sin\,\frac{1}{2}(\alpha + \beta ) = cos\,\frac{1}{2}\,(\alpha - \beta )$

This passes through the point $(ae,0)$ and so:

$e\;cos\;\frac{1}{2}(\alpha + \beta ) = cos\;\frac{1}{2}(\alpha - \beta )$

The meet of the tangents at $P$ and $Q$ is :

$\left(a\;\frac{cos\;\frac{1}{2}(\alpha + \beta )}{cos\;\frac{1}{2}(\alpha - \beta )}\;,\;b\frac{sin\;\frac{1}{2}(\alpha + \beta )}{cos\;\frac{1}{2}(\alpha - \beta )} \right)$

Solution

The $x$ coordinate is :

$\left(a\;\frac{cos\;\frac{1}{2}(\alpha + \beta )}{cos\;\frac{1}{2}(\alpha - \beta )} \right) = \frac{a}{e}$